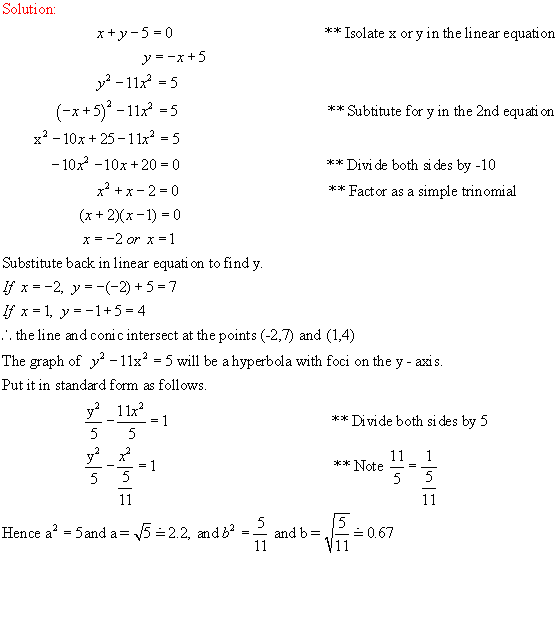|
|
|
|
|
|
|||||||||
|
|
|||||||||||||

UNIT 10 : THE CONICS
LESSON 8: SUMMARY
& REVIEW
LOCUS:
Definition: A locus of points is a set of points which satisfy one or more
given conditions.
A simple
example is a circle. A circle is a
locus (set) of points each of which is a constant distance
from a
fixed point. The fixed point is the centre of the circle and the fixed distance is the radius.
Example 1:
a)
Determine
the locus of points which are 3 units from the origin.
b)
Find
the equation of the locus.
Solution:
a)
The
locus will be a circle, centre the origin and radius of 3 units.
b)
Its
equation can be determined by following these 3 steps
1.
Let
P(x, y) be any point on the locus (circle).
2.
Now
state the condition for P to be on the locus.
P must be always 3 units
from the origin.
![]()
3.
Use
the distance formula to change this statement to equation form.
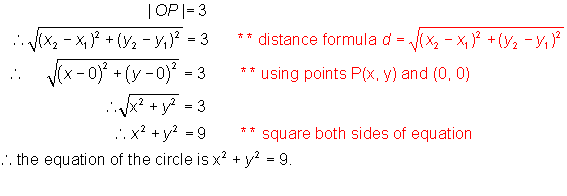

THE CIRCLE:
Definition: A circle is a locus (set) of points each of which is a constant
distance
from a
fixed point. The fixed point is the centre of the circle and the fixed distance is the radius.
![Text Box: Main Ideas:
· The equation of a circle, centre the origin and radius r is:
· The equation of a circle, centre (h, k) and radius r [standard form] is:
· The equation of a circle in general form is;
Use completing the square to put in standard form](./conicsrev_files/image011.gif)
Example 2:
![]()
Solution:
Rewrite as follows and complete the square on both the x
terms and the y terms.
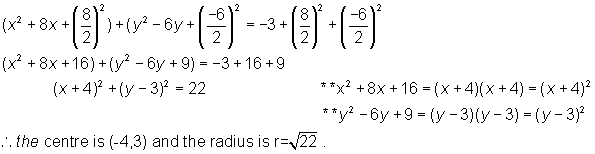
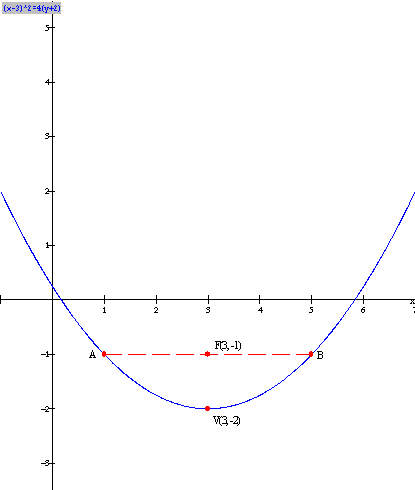
THE PARABOLA:
Definition: Given a fixed point F and a fixed line d in the
plane. A parabola is the locus (set) of
points P in the plane, each of which is equidistant from the
fixed point F (the
focus) and the fixed
line d (the directrix). In the diagram |PF| = |PD| for any point P on the parabola.
The vertex V is the midpoint of the
perpendicular line segment from the
focus F to the directrix d .

![Text Box: Main Ideas for the Parabola:
· |PF| = |PD| for any point P on the parabola.
· The vertex is halfway between the focus and directrix
·
·
·
·
Definitions:
1. A chord is a line segment whose end points are on the curve.
2. A focal chord is a chord that passes through the focus.
3. The focal length is the distance from the vertex to the focus [VF]
4. The length of the focal chord perpendicular to the
axis of symmetry of the parabola is called the
focal width.](./conicsrev_files/image022.gif)
Example 3:

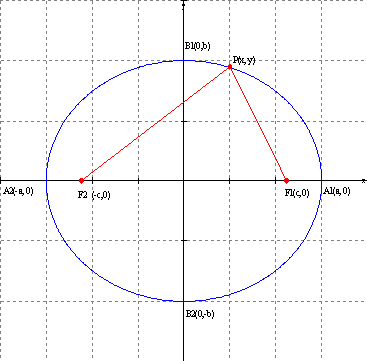
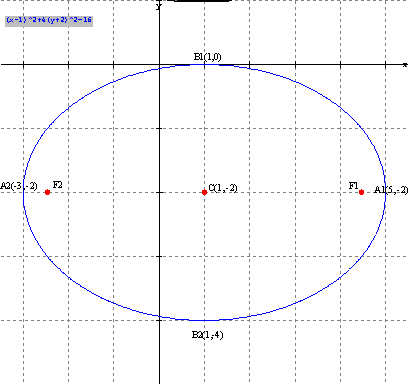
THE ELLIPSE:
Definition: Given two fixed points in the plane F1 and F2. An ellipse is the locus (set) of points P such
that the sum |PF1+ PF2 |
is a constant. The two fixed points are the foci.
The centre is the midpoint of the line segment
joining the two foci (F1F2).

Example 4:

Solution:
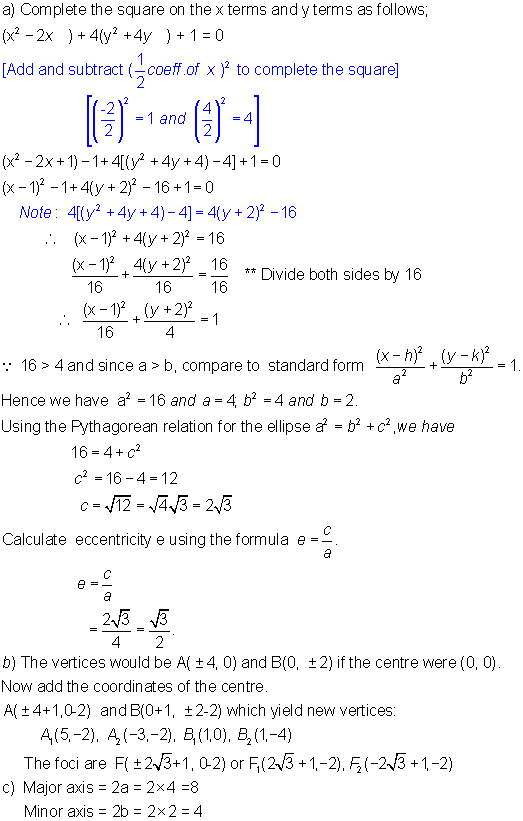
THE HYPERBOLA
:
Definition: Given two fixed points in the plane F1 and F2. A hyperbola is the locus (set) of points P
such that the difference |PF2 PF1 | is a constant. The two fixed points are
the foci.
The centre is the midpoint of the line segment
joining the two foci (F1F2).
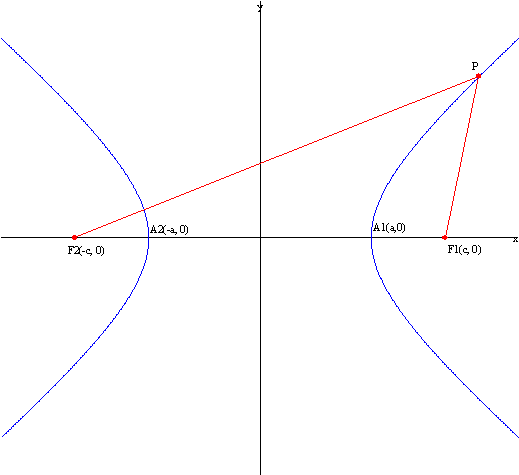
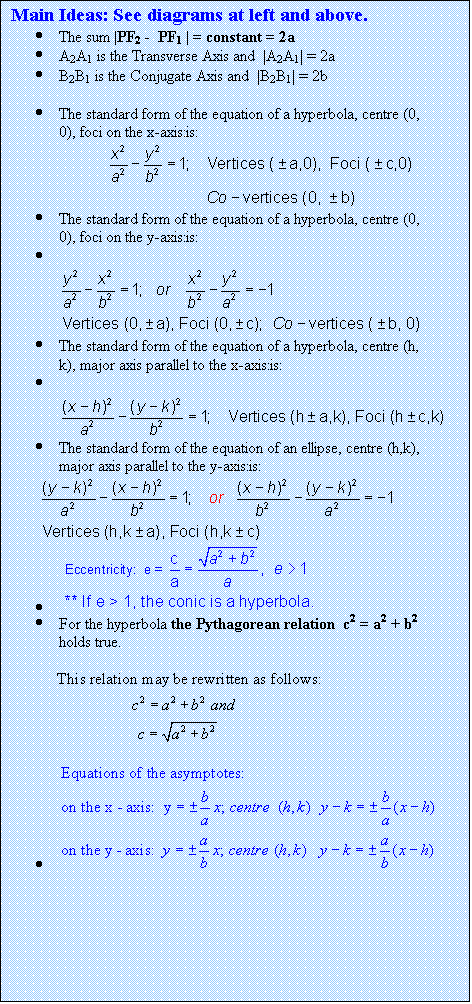
Hyperbola with foci on the
x-axis:
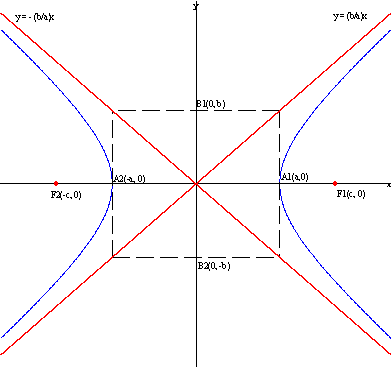
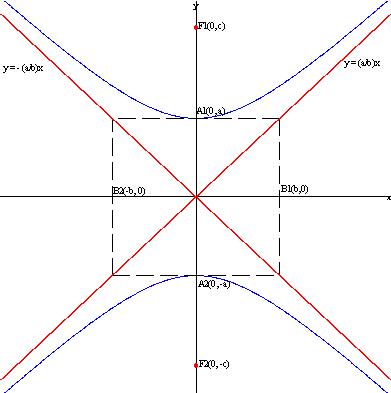
Hyperbola with foci on the y-axis:
Example 5:
Given the hyperbola with equation 9x2 54x 25y2 + 200y 544 = 0.
a) Put the equation in standard form and find the
values of a, b, c, e.
b) Determine the coordinates of the vertices.
c) State the length of the transverse and conjugate axes.
d) Find the equations of the asymptotes.
e) Draw the graph
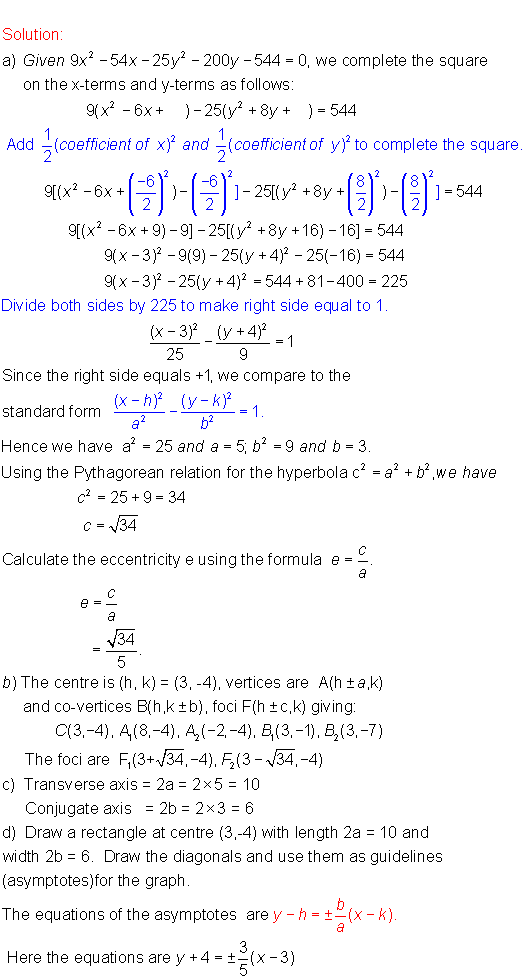
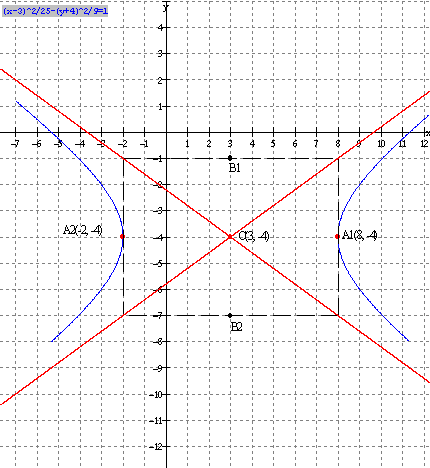
GENERAL FORM OF THE EQUATION OF A
CONIC: ax2 + by2 +2gx + 2fy + c = 0
The type of conic can be determined by the values of the coefficients a and b in the equation according to the following chart.
|
Type of Conic |
Condition on a, b |
|
Circle |
a = b |
|
Parabola |
ab = 0 |
|
Ellipse |
ab > 0 |
|
Hyperbola |
ab < 0 |
INTERSECTION OF LINES
AND CONICS:
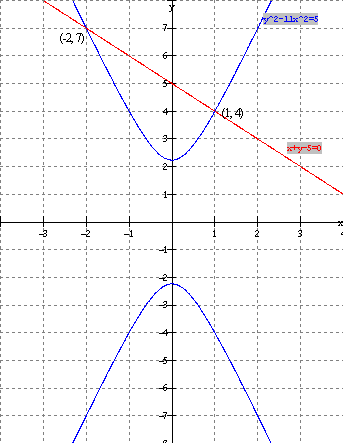
Example 6:
Find the point(s) of intersection of the line x = y 5 = 0 and the conic with equation y2 11x2 = 5.