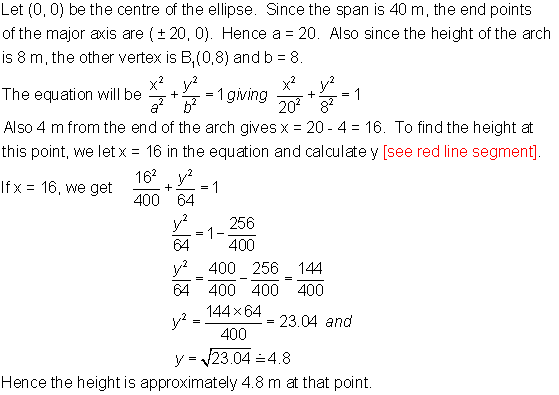|
|
|
|
|
|
|||||||||
|
|
|||||||||||||
|
|
UNIT 10 : THE CONICS
LESSON 3: THE ELLIPSE
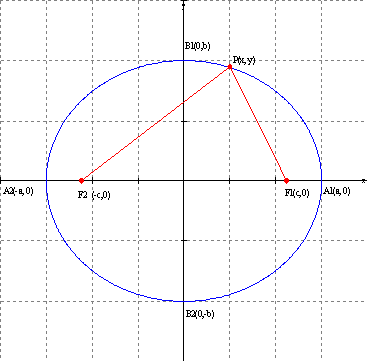
Definition: Given two fixed points in the plane F1 and F2. An ellipse is the locus (set) of points P such
that the sum |PF1+ PF2 |
is a constant. The two fixed points are the foci.
The centre is the midpoint of the line segment
joining the two foci (F1F2).

Example 1:
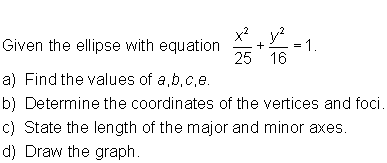
Solution:
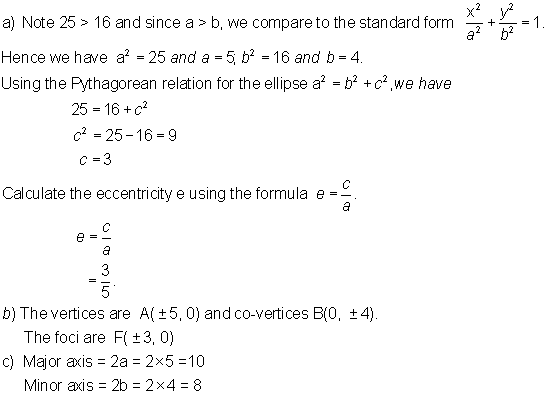

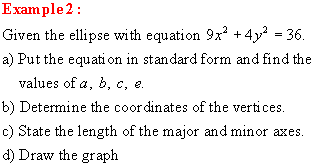

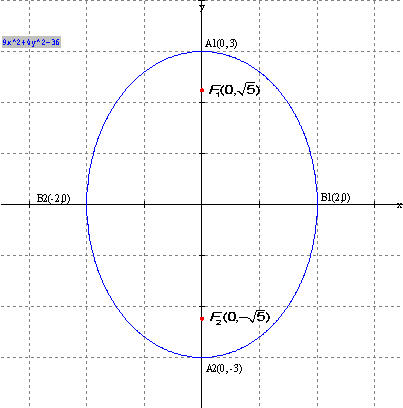
Example 3:
![]()
Solution:
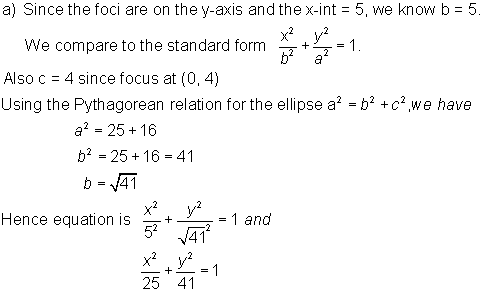
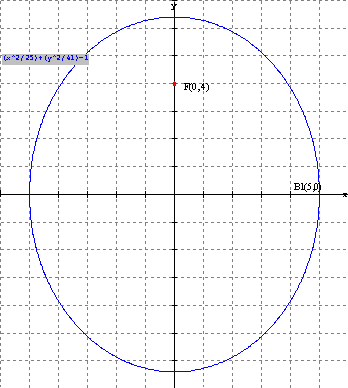
Example 4:

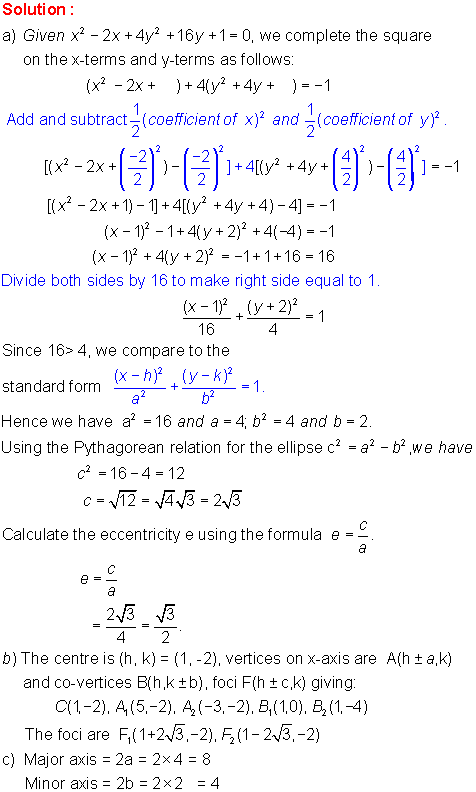
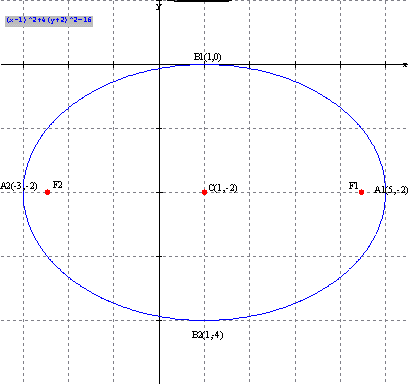
Example 5:
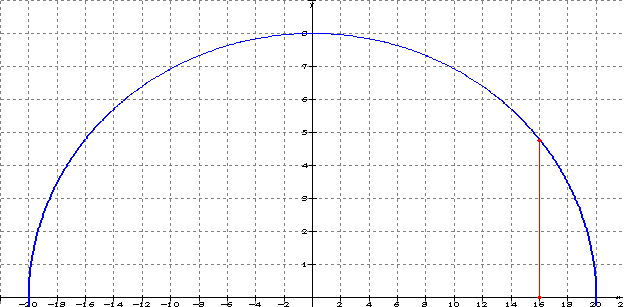
The arch of an overpass is in the shape of a semi-ellipse. If the span of the ellipse (distance between end points of the arch) is 40 m & the height is 8 m,
find the height of the arch at a point 4 m from the end of the arch.
Solution: