|
|
|
|
|
|||||||||
|
|
|||||||||||||
|
|
UNIT 7 : TRIGONOMETRIC FUNCTIONS
LESSON 8
: TRIGONOMETRIC FUNCTIONS REVIEW
Relation
between Radians and degrees:
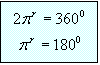
Example 1: Conversions from radian
measure to degree measure
Solution:
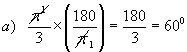
Example 2: Conversions from degree
measure to radian measure
Solution:
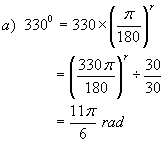
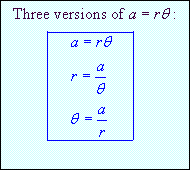
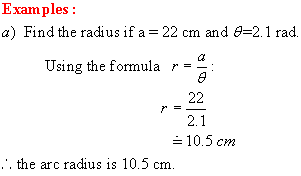
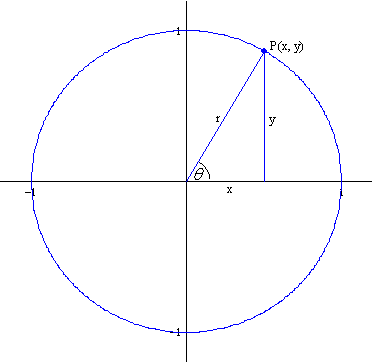
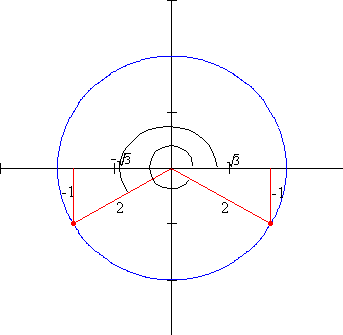
Angles
in Standard Position using {x, y, r}:
Definition: An angle is in standard
position if it has its
vertex at the origin and initial arm along
the positive x-axis. The terminal arm
is found by rotating
the
initial arm about the origin to a terminal position in one of the 4
quadrants. The rotation is positive if
it is in the counter – clockwise direction and negative
if in
the clockwise direction.
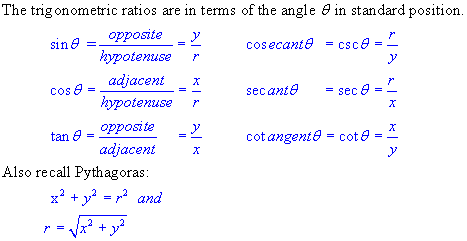
Angles
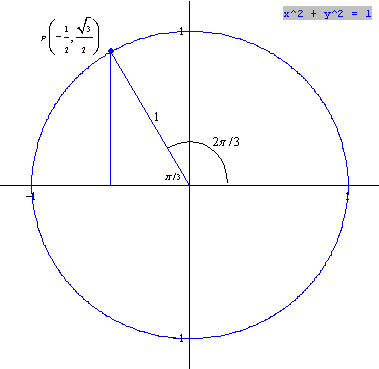
in Standard Position using the Unit Circle:
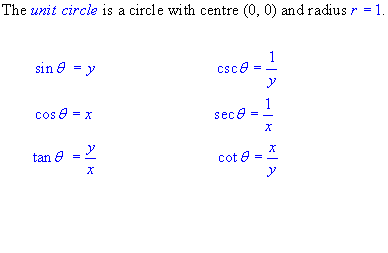
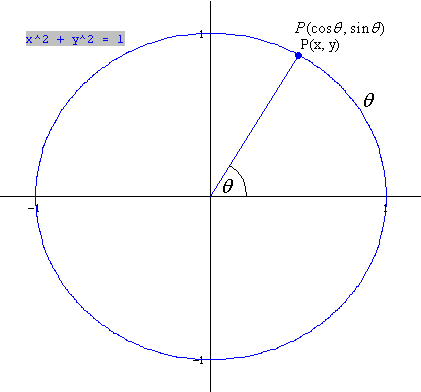
Definition: Coterminal
angles have the same
initial arm and same terminal arm. They
can be found by adding or subtracting 3600 from the given angle.
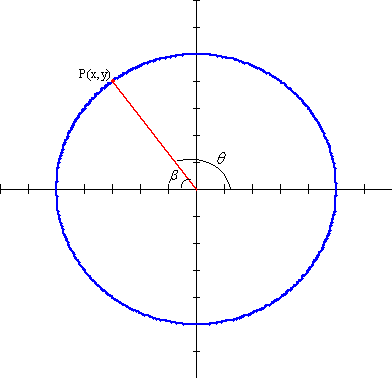
Summary:
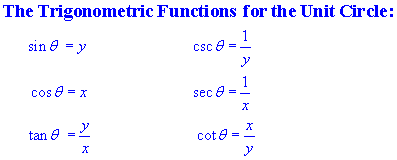
Special
Angles Table:
|
|
|
|
|
|
|
|
|
|
|
|
|
|
1 |
|
|
|
|
|
The Unit Circle for Special Angles
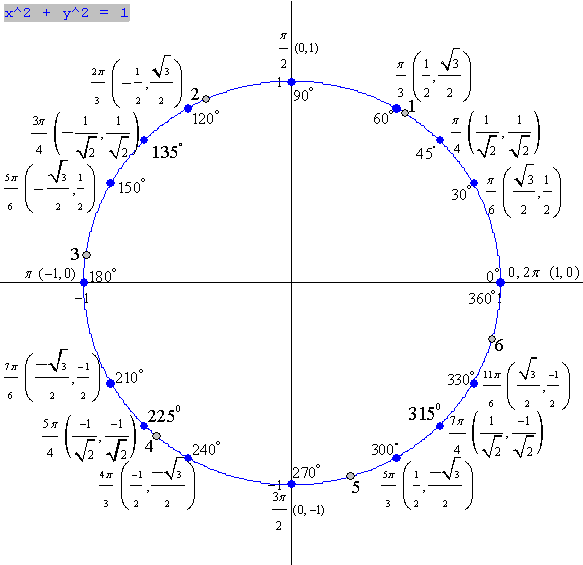
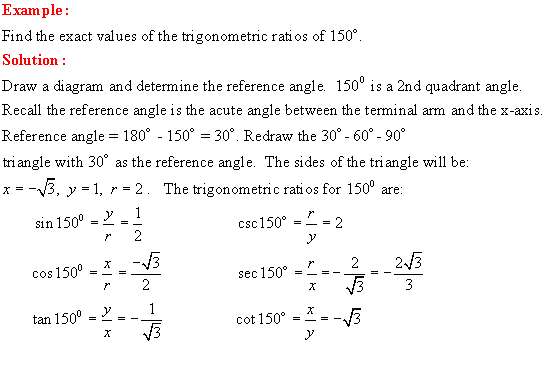
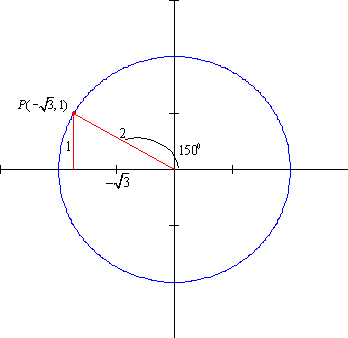
Example :
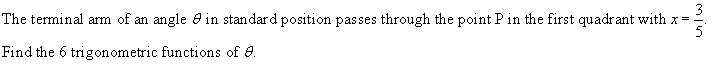
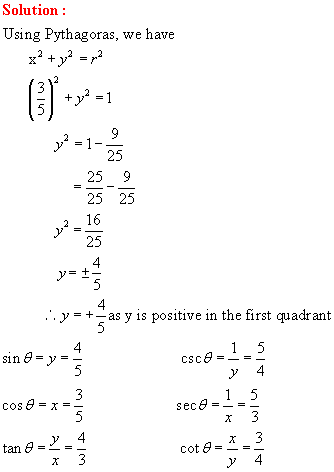
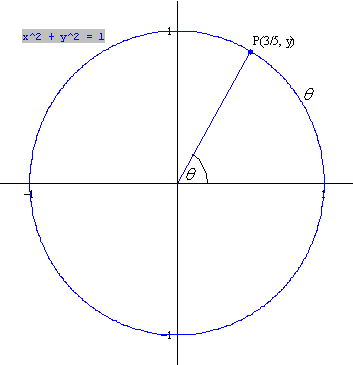
Trigonometric
Functions of Real Numbers Using the CAST RULE & Special Angles Table :
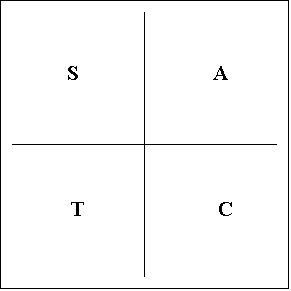The CAST rule:
The CAST
RULE is a memory aid
which tells us the sign of the trig ratios in the various quadrants.
In the
first quadrant ALL are positive. This is denoted using the letter A.
In the
second quadrant, SINE
is positive. This is denoted by
the letter S.
[ the
other two ratios are negative ]
In the
third quadrant, TANGENT
is positive. This is denoted by
the letter T.
[ the
other two ratios are negative ]
In the
fourth quadrant, COSINE
is positive. This is denoted by
the letter C.
[ the
other two ratios are negative ].
Special
Angles Table:
|
|
|
|
|
|
|
|
|
|
|
|
|
|
1 |
|
|
|
|
|
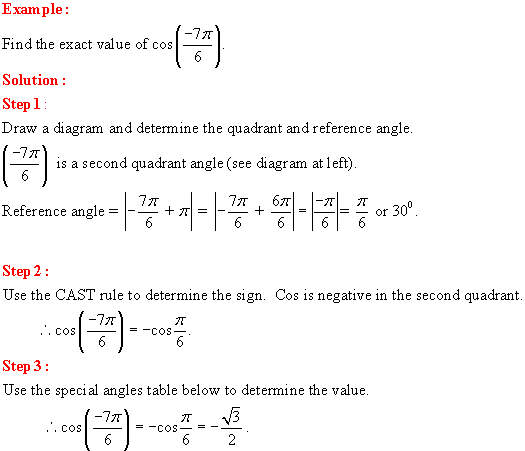
Special Angles Table:
|
|
|
|
|
|
|
|
|
|
|
|
|
|
1 |
|
|
|
|
|
Graphs of Trig. Functions
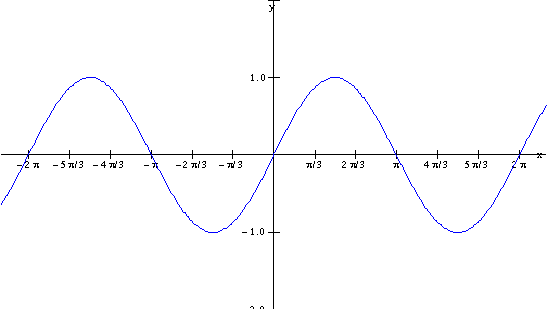
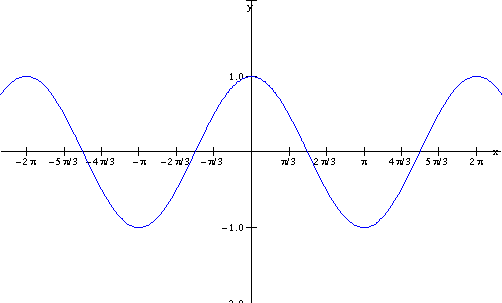
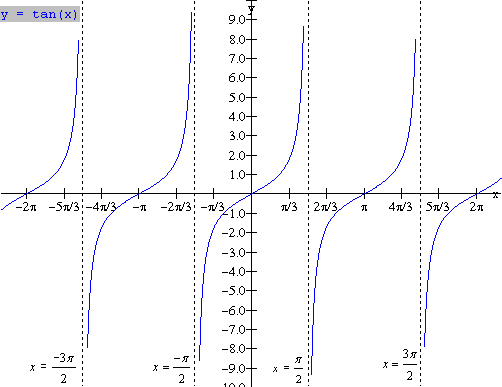
**See lesson 3 for the reciprocal graphs
Combinations
of Transformations : y = a sin k(x
– d) + c and y = a cos k(x – d)
+ c
|
x0 |
0 |
90 |
180 |
270 |
360 |
|
y |
0 |
1 |
0 |
-1 |
0 |
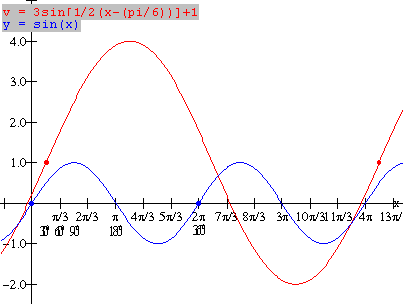
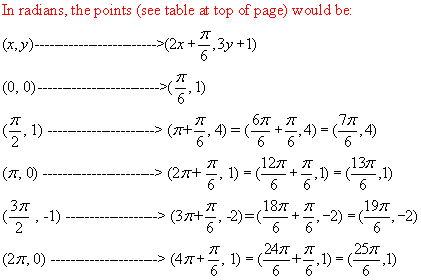
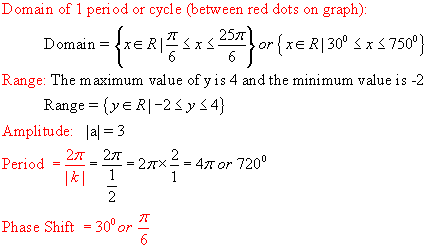
![Text Box: In summary, to graph y = a sin [k(x – d)] + c from the graph of y = sin(x), follow these ideas:
· If a < 0, we have a reflection in the x-axis
· If k < 0, we have a reflection in the y-axis
· If | a | < 1, we have a vertical compression , factor | a |
· If | a | > 1, we have a vertical stretch, factor | a |
·
· If | k | < 1, we have a horizontal stretch, factor 1/k
· If | k | > 1, we have a horizontal compression, factor 1/k
· The value of d gives the horizontal translation (phase shift)
· The value of c gives the vertical translation (shift)](./unitrigrev_files/image143.gif)
Solving
Trig. Equations:
Example:
Solution:
Basic Trigonometric Identities :
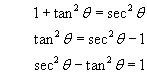
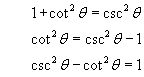
Example :
Proof:
