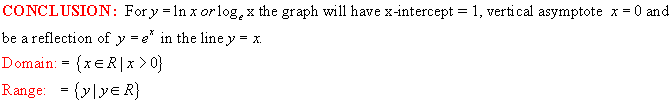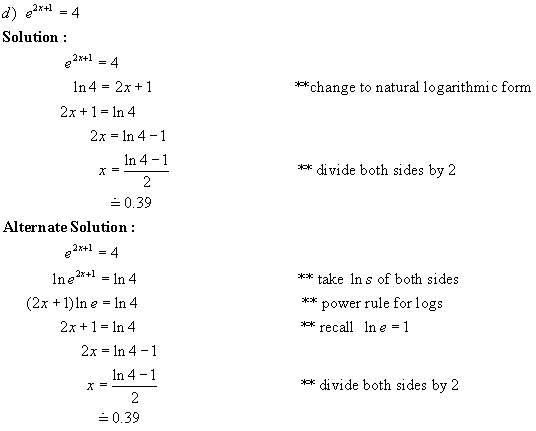New Topic

Exponents

Exponential Equations I

Exponential Functions

The Exponential Function Base e

The Logarithm Function

Logarithmic & Exponential Equations

Applications:Growth & Decay

Review&Test

UNIT 5 : EXPONENTIAL & LOGARITHMIC FUNCTIONS

LESSON 5: THE LOGARITHMIC FUNCTION

Logarithmic Notation:

The exponential statement 2³ = 8 may be rewritten in what is called logarithmic form

as follows: log₂8 = 3 which reads: the logarithm of 8, base 2, equals 3.

Any exponential statement may be rewritten in this form.

Example 1:

Complete the following chart by converting between exponential and logarithmic form.

Exponential Form	Logarithmic Form
3^4 = 81
5^2 = 25
	log232 = 5
	log101000 = 3

Solution:

Exponential Form	Logarithmic Form
3^4 = 81	log381 = 4
5^2 = 25	log525 = 2
2^5 = 32	log232 = 5
10^3 = 1000	log101000 = 3

Note: When we read log₂8, we ask the question “To what exponent must base 2 be raised to give 8?” The answer

is of course 3 and this idea gives rise to the following definition.

Definition: the expression log_ax is defined to mean “ the exponent to which base a must be raised

to give x. The expression reads: “ the logarithm of x, base a.”

Hence log₁₀100 means the exponent to which base 10 must be raised to give 100. The answer is 2, giving the

statement log₁₀100 = 2.

Hence log₃81 means the exponent to which base 3 must be raised to give 81. The answer is 4, giving the

statement log₃81 = 4.

The Logarithmic Function:

Logarithmic functions are the inverses of exponential functions. How does this come about?? See example below.

Table of Values & Graphs:

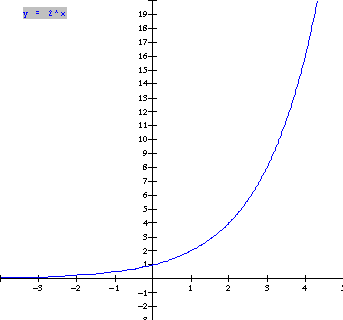
x	y
-3
-2
-1
0	1
1
2
3	8
4	16

Now form the inverse of this function by interchanging x and y in the ordered pairs and the equation.

x	y
	-3
	-2
	-1
1	0
	1
	2
8	3
16	4

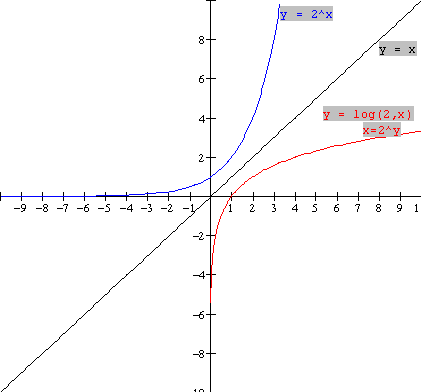
The equation of the inverse is found by interchanging x and y in the equation y = 2^x. This yields x = 2^y. If we put this

equation in logarithmic notation, we obtain the logarithmic function y = log₂x.

The graph is a reflection in the line y = x of the exponential function y = 2^x and is shown in red above.

Table of Values & graph:

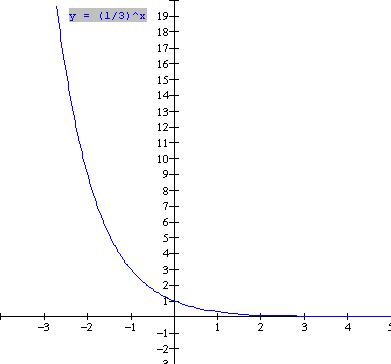
x	y
-3
-2
-1	3
0	1
1
2
3

Now form the inverse of this function by interchanging x and y in the ordered pairs and the equation.

x	y
	-3
	-2
3	-1
1	0
	1
	2
	3

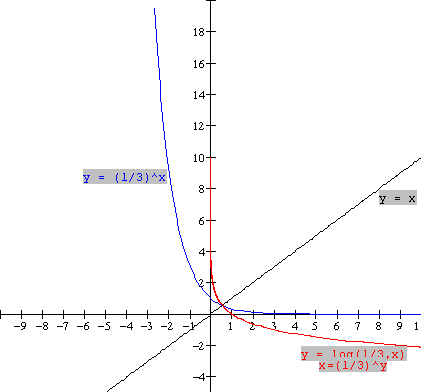
The equation of the inverse is found by interchanging x and y in the equation y = (1/3)^x. This yields x = (1/3)^y. If we put this

equation in logarithmic notation, we obtain the logarithmic function y = log_(1/3)x.

The graph is a reflection in the line y = x of the exponential function y = (1/3)^x and is shown in red above.

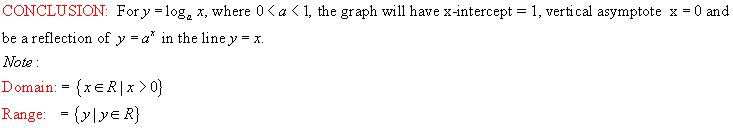
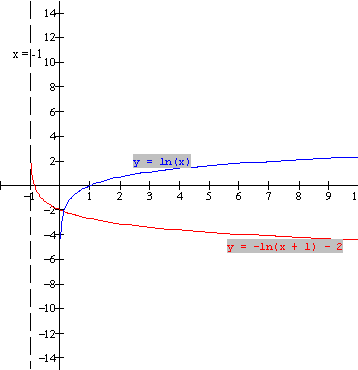
The Natural Logarithmic Function y = log_ex or y = ln x:

x
-3
-2
-1
0	1
1
2
3
4

Now form the inverse of this function by interchanging x and y in the ordered pairs above.

x	y
	-3
	-2
	-1
1	0
	1
	2
	5
	4

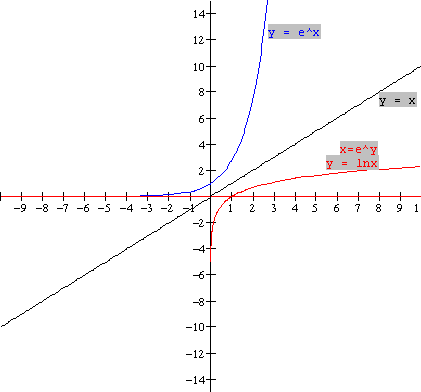
The equation of the inverse is found by interchanging x and y in the equation y = e^x. This yields x = e^y. If we put this

equation in logarithmic notation, we obtain the logarithmic function y = log_ex or y = lnx.

The graph is a reflection in the line y = x of the exponential function y = e^x and is shown in red above.

Return to top of page

Click here to go to homework questions