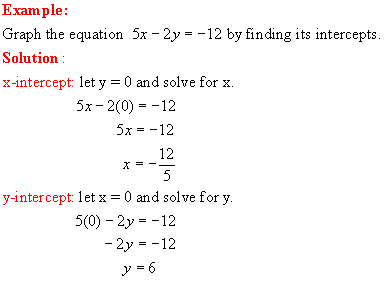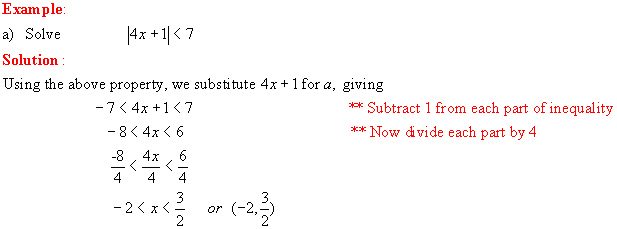|
|
|
|
|
|||||||||||
|
|
|||||||||||||||
UNIT
1 : ALGEBRA PREP
LESSON 10:
LESSON SUMMARY
Review of Basic
Exponent Laws:
|
Rule |
Example |
Explanation |
|
am x an = am+n |
32 x 35 = 37 |
Multiplication Rule - If the bases are the same, add the exponents |
|
|
|
Division Rule - If the bases are the same, subtract the exponents |
|
(am)n
= amn |
(32)3=36 |
Power Rule When taking a power of a power, multiply the exponents |
|
(ab)m
= amam |
(3 x 2)4 = 34
x 24 |
Power of a Product Take each factor in the product to that power |
|
|
|
Power of a Quotient Take numerator and denominator to that power |
Zero
Exponents: Rule: a0 = 1
Examples: 20 = 1;
(-3.4)0 = 1 (x2)0
= 1; Note that 00
is not defined.
Negative
Exponents: Rule: ![]() or
or ![]() or
or 
Examples: ![]() ;
; ![]() ;
; ![]()
Rational
Exponents:
Rule
#1: ![]()
Examples : 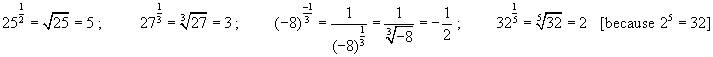
Rule
#2: 
![Text Box: Key Points:
· For fractional exponents, the denominator n gives the index of the root
· The numerator m gives the exponent.
· If n is an odd number, then x can be any real number, positive or negative.
· If n is even, then x must be positive if we are working in the real number system
· Eg. - , [not a Real number since n is even]](./review_files/image029.gif)
Examples : 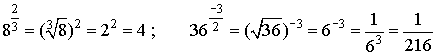
Solving
Inequalities:
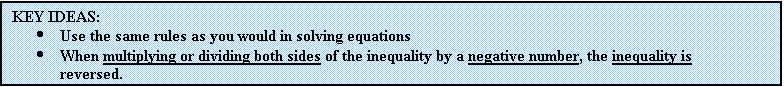
Example 1: Solve 5x 2 < 7x + 8
5x 7x < 8 + 2
-2x < 10
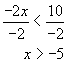 **
Note the inequality reverses when dividing by a negative number
**
Note the inequality reverses when dividing by a negative number
Inequalities
Involving Absolute Value:
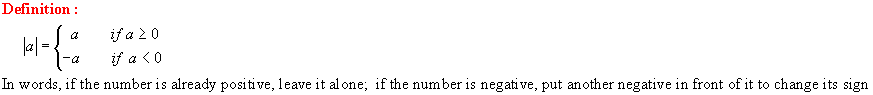
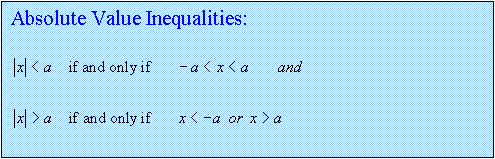
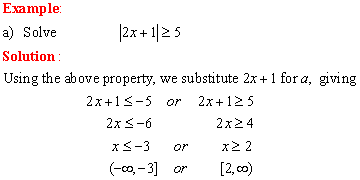
Operations with Radicals:
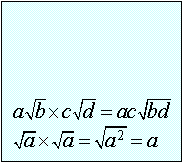

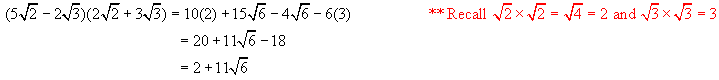
Example:
Simplify

Example: Simplify

Operations with
Polynomials:
Addition and Subtraction:
Example 1: + sign preceding
brackets simply drop the brackets and collect like terms
a) (3x2 2x + 5) + (5x2 3x 6) = 3x2 2x + 5 + 5x2 3x 6 ** drop brackets
= 3x2 + 5x2 2x 3x +5 6 ** Collect like terms
= 8x2 5x 1
Example 2: - sign preceding
brackets multiply each term in the bracket by -1 and collect like
terms
(5x2 3x + 6) (2x2 7x + 8) = (5x2 3x + 6) 1(2x2 7x + 8) ** multiply 2nd bracket by -1
= 5x2 3x + 6 2x2 + 7x - 8
= 5x2 2x2 3x + 7x + 6 8 ** collect like terms
= 3x2 + 4x - 2
Multiplying with Polynomials (Expanding):
Example 1: Monomial x Polynomial
multiply each term in bracket by the monomial
a) 3x(2x2 5x + 7) = -3x(2x2) 3x(-5x) 3x(7) ** multiply each term by -3x
= -6x3 + 15x2 21x
b) 2x(5x 3) 5(2x + 7) = 10x2 6x 10x 35 ** multiply each term in 1st bracket by 2x and 2nd bracket by -5
= 10x2 16x 35 ** collect like terms
Example 2: Polynomial x Polynomial multiply each term in 1st bracket by each term in 2nd bracket
a) (3x + 5)(2x 7) = 3x(2x 7) + 5(2x 7) ** multiply each term in 1st bracket by each term in 2nd bracket
= 6x2 21x + 10x 35 ** expand as in previous example
b) (2x + 3)(3x2 5x 2) = 2x(3x2 5x 2) + 3(3x2 5x 2) ** multiply each term in 1st bracket by each term in 2nd bracket
= 6x3 10x2 4x + 9x2 15x 6 ** expand
= 6x3 x2 19x 6 ** collect like terms
Review of Basic Factoring methods:
1. Common Factoring:
Factor 6x3 15x
Solution: 6x3 15x = 3x(2x2 5) ** Find the HIGHEST COMMON FACTOR for each term --- 3x
** Divide 3x into each term to get the second factor --- 2x2 5
** Check by expanding
2. Difference of Squares: Formula -- a2 b2 = (a b)(a + b)
Factor 49x2 64y2
Solution: 49x2 64y2 = (7x 8y)(7x + 8y)
Factor x2 9y2
Solution: x2 9y2 = (x 3y)(x + 3y)
3. Simple Trinomials: Form x2 + bx + c [Coefficient of x2 is 1]
a) Factor x2 + 5x + 6
Solution: Recall x2 + 5x + 6 = (x + __ )(x + __ ) ** We need two numbers that multiply to +6 and add to +5
** Check all pairs of factors of 6: {1, 6} adds to 7
{2, 3} adds to 5
Hence x2 + 5x + 6 = (x + 3 )(x + 2) ** Check by expanding
4. Hard Trinomials: Form ax2 + bx + c [Coefficient of x2 does not equal 1]
a) Factor 6m2 5m 4
Solution:
We use the method of decomposition (although there are other methods)
We decompose the middle term -5m into two parts using the two clues:
Multiply
to (6)(-4) = -24 and
Add to -5
Strategy: List all pairs of factors of -24 and find the pair that adds to -5 ** {1, 24} cannot obtain -5 with these two factors; 1+24=25; 1-24= -23
** {2, 12} cannot obtain -5 with these two factors
** {3, 8} -8 + 3 = -24 Choose these two factors
Hence 6m2 5m 4 = 6m2 8m + 3m 4 ** -5m broken into two parts -8m + 3m
= 2m(3m 4) + 1(3m 4) ** Group by twos and common factor
= (3m 4)(2m + 1) ** check by expanding
Simplifying Rational Expressions:
·
Factor the numerator and denominator if possible
·
Reduce to lowest terms by dividing out common factors
·
State any restrictions remember the denominator cannot equal 0.
![]() =
= ![]()
Restrictions:
![]() and
and ![]()
Multiplying Rational
Expressions:

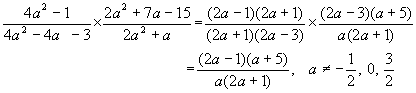
Dividing Rational
Expressions:


Adding and Subtracting Rational Expressions:

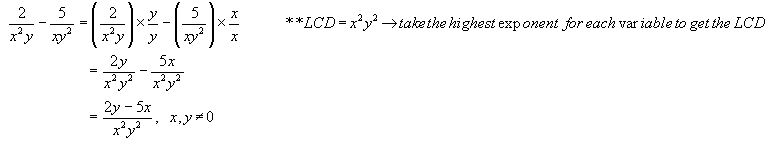

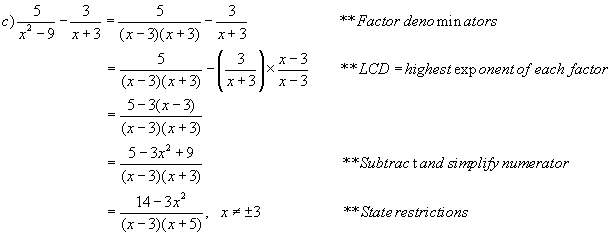
Slope of a Line:
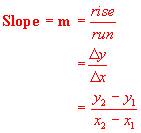

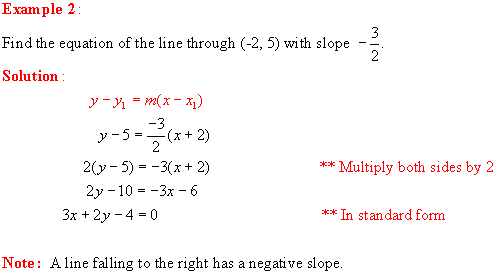
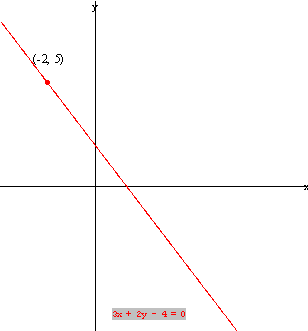
Slope y-intercept Form of the Equation of a Line:
![]()
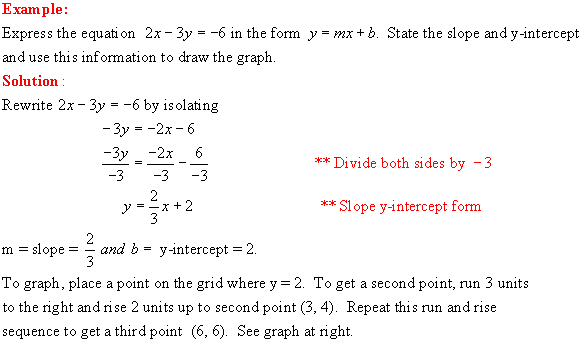
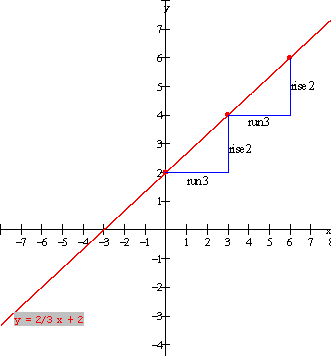
Graphing Lines Using the Intercept Method: